Knowing the combination of digits that can fit into a killer sudoku cage isn’t just a useful technique, sometimes it is absolutely necessary in order to solve or start a puzzle. Remembering common unique combinations is essential if you want to improve your time for solving killer sudoku puzzles. Unless you have a photographic memory though, you probably won’t memorise all of them, which is why this cheat sheet can be handy.
As well as cell cage combinations, we've included required digits further down. Some cells always require particular digits, regardless of the number combination that goes into them. Knowing these is a great way to eliminate candidate numbers from blocks, rows, and columns.
Is it cheating? We call it a cheat sheet, but is it really cheating? Only you can decide! Our view is that a reference like this is no more cheating than using a dictionary to check your spelling. For us, puzzles like killer sudoku are all about the logic and not an exercise in memory or recall.
New to killer sudoku? Be sure to check out our Killer Sudoku From Scratch tutorial.
Love logic puzzles? Be sure to check out Puzzle Weekly – our free weekly collection, delivered to your inbox every Monday.
Click here to find out more, and to get your free subscription.
These are all possible combinations of digits for a given cage size and sum. Bolded sums have only one combination.
3 — 12
4 — 13
5 — 14 23
6 — 15 24
7 — 16 25 34
8 — 17 26 35
9 — 18 27 36 45
10 — 19 28 37 46
11 — 29 38 47 56
12 — 39 48 57
13 — 49 58 67
14 — 59 68
15 — 69 78
16 — 79
17 — 89
6 — 123
7 — 124
8 — 125 134
9 — 126 135 234
10 — 127 136 145 235
11 — 128 137 146 236 245
12 — 129 138 147 156 237 246 345
13 — 139 148 157 238 247 256 346
14 — 149 158 167 239 248 257 347 356
15 — 159 168 249 258 267 348 357 456
16 — 169 178 259 268 349 358 367 457
17 — 179 269 278 359 368 458 467
18 — 189 279 369 378 459 468 567
19 — 289 379 469 478 568
20 — 389 479 569 578
21 — 489 579 678
22 — 589 679
23 — 689
24 — 789
10 — 1234
11 — 1235
12 — 1236 1245
13 — 1237 1246 1345
14 — 1238 1247 1256 1346 2345
15 — 1239 1248 1257 1347 1356 2346
16 — 1249 1258 1267 1348 1357 1456 2347 2356
17 — 1259 1268 1349 1358 1367 1457 2348 2357 2456
18 — 1269 1278 1359 1368 1458 1467 2349 2358 2367 2457 3456
19 — 1279 1369 1378 1459 1468 1567 2359 2368 2458 2467 3457
20 — 1289 1379 1469 1478 1568 2369 2378 2459 2468 2567 3458 3467
21 — 1389 1479 1569 1578 2379 2469 2478 2568 3459 3468 3567
22 — 1489 1579 1678 2389 2479 2569 2578 3469 3478 3568 4567
23 — 1589 1679 2489 2579 2678 3479 3569 3578 4568
24 — 1689 2589 2679 3489 3579 3678 4569 4578
25 — 1789 2689 3589 3679 4579 4678
26 — 2789 3689 4589 4679 5678
27 — 3789 4689 5679
28 — 4789 5689
29 — 5789
30 — 6789
15 — 12345
16 — 12346
17 — 12347 12356
18 — 12348 12357 12456
19 — 12349 12358 12367 12457 13456
20 — 12359 12368 12458 12467 13457 23456
21 — 12369 12378 12459 12468 12567 13458 13467 23457
22 — 12379 12469 12478 12568 13459 13468 13567 23458 23467
23 — 12389 12479 12569 12578 13469 13478 13568 14567 23459 23468 23567
24 — 12489 12579 12678 13479 13569 13578 14568 23469 23478 23568 24567
25 — 12589 12679 13489 13579 13678 14569 14578 23479 23569 23578 24568 34567
26 — 12689 13589 13679 14579 14678 23489 23579 23678 24569 24578 34568
27 — 12789 13689 14589 14679 15678 23589 23679 24579 24678 34569 34578
28 — 13789 14689 15679 23689 24589 24679 25678 34579 34678
29 — 14789 15689 23789 24689 25679 34589 34679 35678
30 — 15789 24789 25689 34689 35679 45678
31 — 16789 25789 34789 35689 45679
32 — 26789 35789 45689
33 — 36789 45789
34 — 46789
35 — 56789
21 — 123456
22 — 123457
23 — 123458 123467
24 — 123459 123468 123567
25 — 123469 123478 123568 124567
26 — 123479 123569 123578 124568 134567
27 — 123489 123579 123678 124569 124578 134568 234567
28 — 123589 123679 124579 124678 134569 134578 234568
29 — 123689 124589 124679 125678 134579 134678 234569 234578
30 — 123789 124689 125679 134589 134679 135678 234579 234678
31 — 124789 125689 134689 135679 145678 234589 234679 235678
32 — 125789 134789 135689 145679 234689 235679 245678
33 — 126789 135789 145689 234789 235689 245679 345678
34 — 136789 145789 235789 245689 345679
35 — 146789 236789 245789 345689
36 — 156789 246789 345789
37 — 256789 346789
38 — 356789
39 — 456789
28 — 1234567
29 — 1234568
30 — 1234569 1234578
31 — 1234579 1234678
32 — 1234589 1234679 1235678
33 — 1234689 1235679 1245678
34 — 1234789 1235689 1245679 1345678
35 — 1235789 1245689 1345679 2345678
36 — 1236789 1245789 1345689 2345679
37 — 1246789 1345789 2345689
38 — 1256789 1346789 2345789
39 — 1356789 2346789
40 — 1456789 2356789
41 — 2456789
42 — 3456789
36 — 12345678
37 — 12345679
38 — 12345689
39 — 12345789
40 — 12346789
41 — 12356789
42 — 12456789
43 — 13456789
44 — 23456789
45 — 123456789
These are digits that must be present somewhere within a cage for a given sum.
8 — 1
22 - 9
12 — 1,2
13 — 1
27 — 9
28 — 8,9
17 — 1,2,3
18 — 1,2
18 — 1,2
19 — 1,2
20 — 1,2
21 — 1
31 — 9
32 — 8,9
33 — 7,8,9
23 — 1,2,3,4
24 — 1,2,3
25 — 1,2
26 — 1
34 — 9
35 — 8,9
36 — 7,8,9
37 — 6,7,8,9
30 — 1,2,3,4,5
31 — 1,2,3,4
32 — 1,2,3
33 — 1,2,6
34 — 1
36 — 9
37 — 8,9
38 — 7,8,9
39 — 3,6,7,8,9
40 — 5,6,7,8,9
If you’ve never played killer sudoku but want to get started, or if you’ve taken a look at a killer sudoku grid and wondered how on Earth you’re supposed to begin solving it, then you’ve come to the right place.
Killer sudoku is like regular sudoku but with added mathematics. Unlike the classic game, there’s arithmetic involved. If that’s not your thing, killer sudoku probably isn’t for you!
A quick word of caution before we get started: killer sudoku is very much based on regular sudoku. You really need to know how to play the classic version before tackling this kind of puzzle. So if you’ve never solved a sudoku puzzle before, I would recommend reading through our Sudoku From Scratch guide, and doing some simple puzzles first (there are some free ones to download included in our guide).
With that said, let’s dive in and look at a killer sudoku grid, and the rules that govern the puzzle. Here’s an easy grid to start us off — it’s a 1-star grid from our Pocket Killer Sudoku range.
There are lots of things that are familiar about this grid if you are used to sudoku:
There are also a couple of very obvious differences between a killer sudoku puzzle and the regular variety.
The first rule of killer sudoku is the same as sudoku:
To this we add a new rule:
There’s the arithmetic; every killer sudoku puzzle involves a bit of adding up.
Looking back at our example grid above, we can see that the yellow cage has a little 8 written in the top cell. That means that the three numbers that go in that cage must add up to eight.
Whether you want to do your killer sudoku arithmatic in your head, or with the aid of a calculator, is up to you. But beware: any mistakes in adding up clue numbers will lead to errors that make the puzzle impossible to solve!
Solving killer sudoku puzzles requires a number of techniques. Some of those are the same as regular sudoku. Hidden and naked singles, single free cells, matching pairs and so on, all work exactly the same. But of course to use those we need to have some cells filled in, and when we begin a killer sudoku there aren’t any. So we can’t begin solving a killer sudoku puzzle the same way we would with a regular grid. We have to find another means of making headway. We need some specialist techniques, and that’s what we’re going to learn now.
When we add up all the digits from 1 to 9, we get 45. We can therefore deduce that the sum of every row in a killer sudoku grid must be 45, that the sum of every column must be 45, and the sum of every block must also be 45. How does this help us solve a puzzle? Consider our example grid again:
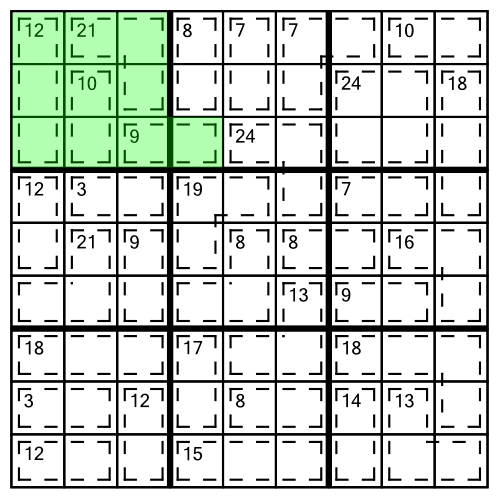
Looking at the top left block we notice that all but one of the cages are fully contained within it. The 9 cage spills over into the next block. We know that the sum of the block must be 45. We can add up the clue numbers (12+21+10+9) to get 52. That’s bigger than 45 - the maximum sum possible in a block. Therefore everything over and above 45 must fit into the single cell that spills out of the block. 52 minus 45 is 7, so that cell must contain a 7. Which in turn means the other cell in the cage has to be a 2:
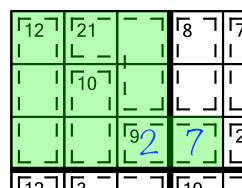
Naturally we can use the same technique on rows and columns as well as blocks.
Sometimes cages don’t spill out out an area, they spill into one. Here’s an example from another 1-star grid:
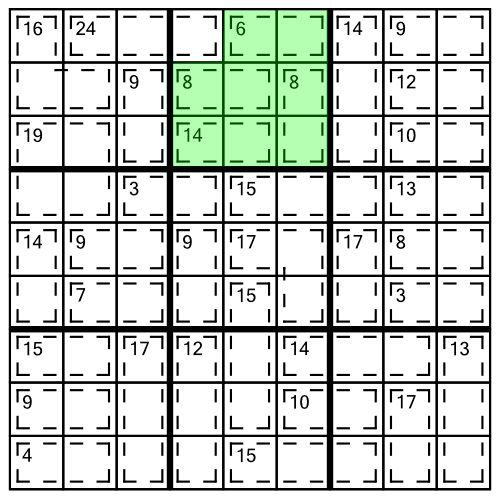
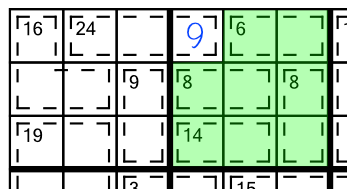
The principle is exactly the same. We add up the sum of the cages wholly contained within the block, to work out the value of the cell that has spilled into it.
6 + 8 + 8 + 14 = 36. We need all the cells in the block to add up to 45, so the cell that has spilled into it (the only one not shaded green), must contain a 9 (45 - 36 = 9):
Looking for places to apply this 45 rule is a good way to start a puzzle because it allows us to fill in some cells very quickly. Our example puzzles here are 1-star (easy) level. Harder puzzles won’t give up their secrets so easily. However, it’s worth keeping an eye out for places to apply this rule as you progress through a puzzle, because as cells get filled in, they can be used in the sum calculations, opening up more places to use this simple technique.
We can take the 45-rule further by applying it to multiple columns, rows, or blocks simultaneously:
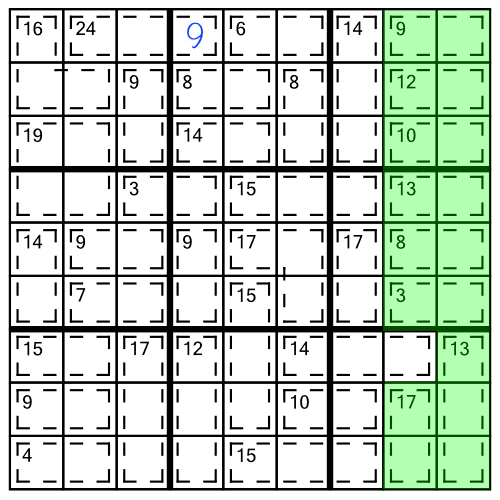
Neither of these columns alone are candidates for the 45 rule, but by combining them we find we have a single cell spilling inside. Working out its value is simple. We know the sum of the highlighted columns must be 90 (2 columns x 45). If we add up the clue numbers we get 85. Therefore the cell spilling into the columns must contain a 5, because 90 - 85 is 5.
Here’s another example, this time combining three blocks together:
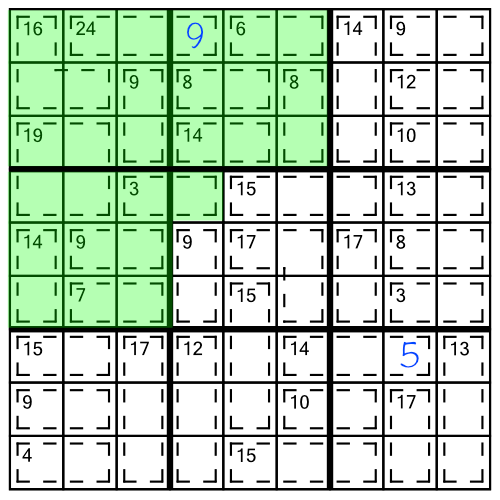
Adding all the clue numbers together gives us 137. As we have three blocks, we know the total should be 135 (3 x 45), so the extra 2 must go in the cell spilling out:
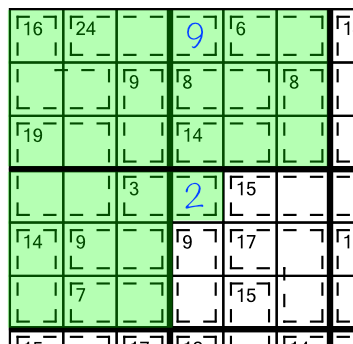
That in turn tells us we can put a 1 in the second and last remaining cell in that cage:
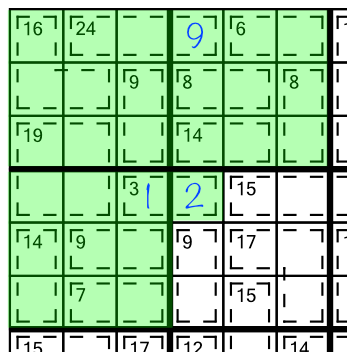
For any given cage, there are only a limited number of digits that can legitimately add up to its clue number. This block contains a cage with an 3 in it, and it’s made of two cells. For brevity, we notate this as a 3(2) cage.
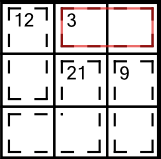
The only numbers that add up to 3 are 1+2. Therefore one of the cells must contain a 1 and the other must contain a 2. On its own this information doesn’t allow us to solve either cell definitively, but it narrows them down to two possible digits each, and we can write those in as little numbers, just like in sudoku:
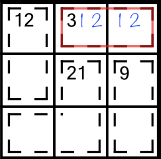
We know from sudoku that matching pairs like this are very useful. This one allows us to eliminate the numbers 1 and 2 from the rest of the row and and the rest of the block.
Now let’s consider the example of an 8(2) cage (so that’s a two cell cage with an 8 written in it). Here are the possible combinations of numbers that could go in those cells:
Why not 4 and 4? Because no two cell cage could possibly contain the same digit twice — it would mean putting the same digit twice into a column or row.
Knowing that each cell in an 8(2) cage could contain a 1, 2, 3, 5, 6 or 7 isn’t very useful. But other cages are much more restricted - as was the case with our 3(2) example earlier. To save you working out other combinations yourself, here are all the two-cell cages that can only contain two digits:
If you want to see the limited sum combinations for larger cages, take a look at our killer sudoku cheat sheet.
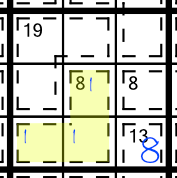
There are certain sum combinations that always contain certain digits. For example, our yellow highlighted 8(3) cage right at the top of the page could comprise any of the following:
Knowing that any of the three cells in the cage could contain a 1, 2, 3, 4 or 5 is not very handy. But knowing that one of them must contain a 1 is much more useful. It allows us to write a little 1 into each of those three cells, thus eliminating it from the rest of the block.
We’ve put the required digit sums into our cheat sheet too, to save you some more time. As with limited sum combinations, learning some of these by heart is a sure way to speed up your solving skills.
We can push a puzzle a long way just by combining the techniques we have looked at so far, along with using what we already know about sudoku. Let’s work through a quick example. Consider the middle block of this right-hand stack:
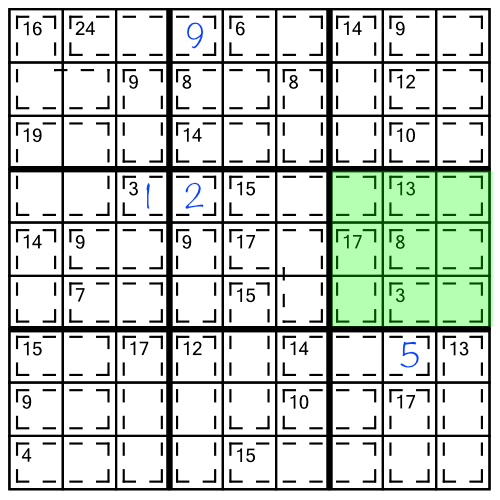
The first thing we can and should do, is work out the contents of the cell from the 15(3) cage that is spilling inside this block. Adding up the cages wholly contained in our block yields 41, so the intruding cage's cell must contain the missing 4 to bring us up to the requisite 45.
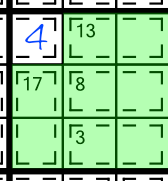
Next, based on what we know about limited sum combinations, we deduce that the 17(2) cage can only contain an 8 and a 9. And we can also work out that the 3(2) cage can only contain a 1 and a 2. Look what happens when we write in those little numbers:
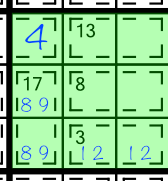
Knowing that this block already has the digits 1, 2, 4, 8 and 9 taken care of, reduces the possible candidates for the other cells. So whereas an 8(2) cage could normally take candidates 1,2,3,5,6 and 7, here we can reduce that list to 3,5,6 and 7. And in fact if we zoom out and look at the stack again, we notice that there’s already a 5 in the middle column, so we can reduce the options even further in half of the cage.
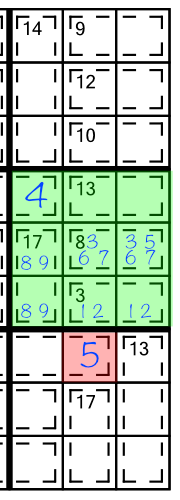
The same logic applies to the 13(2) cage. Normally it could hold a 4,5,6,7,8, or 9. Given what we know must already be in the 17(2) and 3(2) cages, and the cell that’s already filled in, we can reduce those candidates to 5,6, and 7. And again, the 5 already in the column reduces the options further in the first cell in that cage:
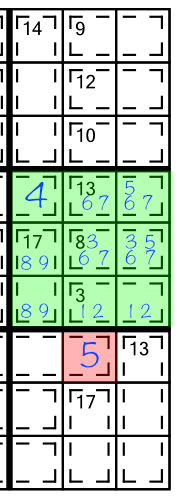
Working across the puzzle in this way, combined with regular sudoku techniques, is enough to solve easy-intermediate puzzles like the examples presented here.
The key to solving easy killer sudoku puzzles is to use all the tools we’ve covered, to eliminate possible candidates. Often you will find that you can reduce and reduce the possibilities within a cage so far that you solve one cell in the cage. That in turn reduces the possible candidates for other cells within the same cage, and so on.
Remember to always look at the repercussions of each cell you solve — in killer sudoku even more so than in regular sudoku, solving a single cell can have huge repercussions that ripple out across the whole puzzle.
What starts off as a seemingly impenetrable grid, can very quickly evolve into something that becomes easy to solve as you combine the killer sudoku sum clues with regular sudoku techniques.
If you want to practice solving 1-star puzzles, we’ve put together a set you can download and print out below, as well as the solutions to check your answers. And of course, we have a full range of high-quality killer sudoku books, including our popular A Year of Killer Sudoku - with a new puzzle every day for a year.
Killer Sudoku Practice — Grids
Killer Sudoku Practice — Solutions
Right click or long-tap and Download Linked File or click or tap to open in a new window then choose Print from your browser.