We've got another new puzzle for you this week: Arrows. It's quite different to anything we've published before, and rather addictive – you have been warned! Also this week, Killer Sudoku, Hidato, and all the usual features including:

Trilogy is a pure logic puzzle that uses symbols on a square grid. The aim is to fill the empty cells on the grid with the correct missing symbols.
In a hurry? Jump to: Rules / Solving / Tips / Worked Example / Download Free Puzzles / Trilogy Books
Here’s what a small Trilogy puzzle looks like:
There are three symbols used in the puzzle: circles, squares, and triangles. There are only two rules to remember:
Here’s what the earlier example looks like once solved:
Solving these puzzles requires the application of logic. There are lots of patterns you can use to help you, and the more puzzles you do, the more easily you’ll spot the patterns.
These patterns are all “forced moves” – they allow you to immediately place a new symbol on the grid. Easier puzzles have more of these patterns, but they’ll help with harder puzzles too; as you add more symbols, more patterns will emerge.
In this tutorial we will use the notation A, B, C, and x, where A, B and C stand in for the three symbols and x is the cell we are trying to solve.
There are two kinds of pattern. The easiest is a simple row of four cells with a gap, allowing you to place a symbol:
AxCC pattern. In this case, the x must be the same as the A symbol. It can’t be B because otherwise we’d have (ABC)C, which isn’t allowed, and it can’t be C because otherwise we’d have A(CCC), which is also not allowed.
In this example, A is a square and C is a circle, so the empty (x) cell must be a square.

ABxB pattern. In this case the empty cell must contain the A symbol, because (ABC)B isn't allowed, and A(BBB) is not allowed.
In this example, A is a square and B is a triangle, so the empty (x) cell must be a square.

These patterns are easy to spot as you can quickly look for doubles on the grid (two symbols the same next to each other), and doubles with a gap between and another symbol next to them.
Next, you can look for intersection patterns. These are patterns where the empty cell we are trying to solve is at the intersection of two patterns. The simplest intersections are a confluence of doubles. For example, if you have AAx in one direction, and BBx in another, x must obviously be C, otherwise we’d be making a triple, which isn’t allowed.
In this example, we have two circles together vertically, forming an AAx, intersecting with two triangles together diagonally, forming a BBx. The intersecting cell (x) must be a C to avoid three in a row. Therefore, in this case it would be a square.
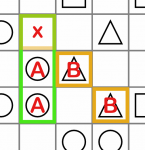
There are lots of these patterns to find in Trilogy. The following table lists some common intersections and what they resolve to. It should be fairly easy to work out why the answer is what it is in each case.
| Solves To: | ||
| AAx | BBx | C |
| AAx | ABx | B |
| ACx | BAx | A |
| AxA | ABx | B |
| AxC | ABx | A |
These are not necessarily all the possible patterns – they are the most common, and a good starting place. As you get deeper into this fascinating puzzle, you will find more that you can add to your arsenal.
Here are some further tips to help you solve Trilogy:
Let’s put all of that into practice and solve a simple puzzle. This is not intended to show the most efficient way of solving this puzzle. The purpose of the example is to demonstrate the strategies we can use to solve Trilogy, and is just one way of getting to the solution.
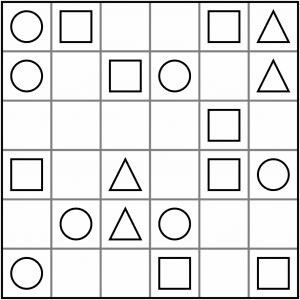
This is the puzzle we are going to solve. We’ve deliberately chosen a simple one to keep this tutorial manageable. The same concepts apply whatever the size of the grid, though.
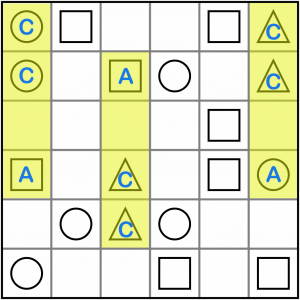
Let’s begin by looking for the easiest patterns. The first of those is the AxCC. There are three of those in this puzzle, all in the vertical plane. We know that the empty cell in this pattern must be the A symbol. If we put a C in there, we’d have three Cs in a row, which isn’t allowed. And if we put a B in there, we would have A, B and C in a row, which also isn’t allowed. So from the left, the first empty cell must be a square, the second also a square, and the third one, on the right edge, must be a circle.
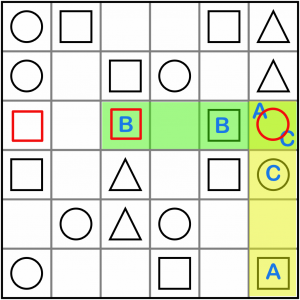
Filling in those three symbols has created a a new AxCC pattern (in yellow), so we know the empty cell must be a square. It’s also given us an ABxB pattern (green). This must solve to A, for exactly the same reason as before. So the empty box in the green area must be a circle.
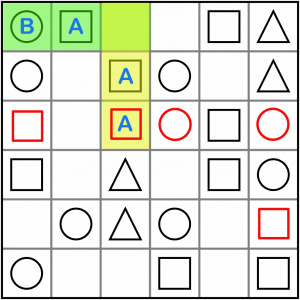
We’ve exhausted the simple gap patterns for now. Let’s look for intersections. The easiest to spot are the doubles (it’s quick to find two symbols the same on the grid). Here’s one example, right at the top. We have an AAx intersecting with a BAx. We know this must solve to B, because if we tried to put an A in the intersecting cell we’d have AAA which isn’t allowed, and if we tried to put a C in there, we’d have BAC which is also not allowed. So the empty cell must contain a circle.
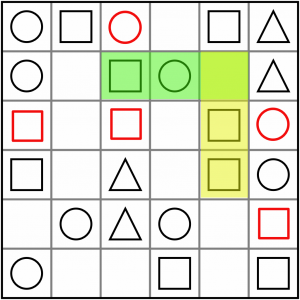
Here’s another, almost the same. This time it’s ABx / AAx, but it solves to the same solution for the same reason. We have to put a circle in the intersecting cell.
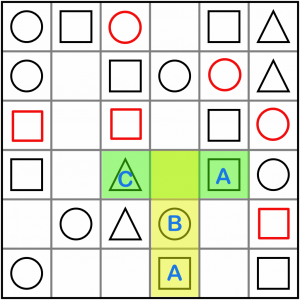
Here’s a different kind of intersection. This is an AxC / ABx. From our table above, we know it resolves to A. That’s because if we tried to put a B in the intersecting cell, we would be creating an ABC run in the green area, and if we put a C in there, we’d be creating an ABC run in the yellow one. A is the only option. The empty cell contains a square.
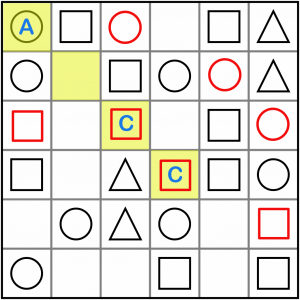
This is an easy puzzle, and there are lots of intersections and patterns appearing all over the place. Let’s look at this AxCC for no other reason than because it’s a diagonal, and we haven’t done any of those yet! It’s important to remember to check the diagonals, particularly when puzzles get harder, as they add two extra dimensions and can often be the key to unlocking the grid.
Being an AxCC pattern, we know the empty cell solves to A, so it’s a circle.
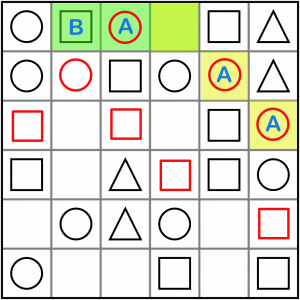
We can do intersections on diagonals as well. Here’s another AAx / BAx, with the AAx on the diagonal. We know it solves to B, so the empty cell at the intersection must be a square.
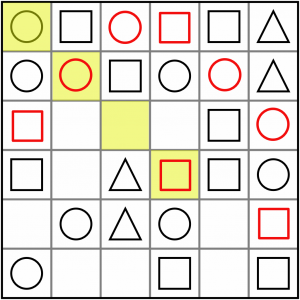
Here’s another diagonal, an AxCC. It must be a square.
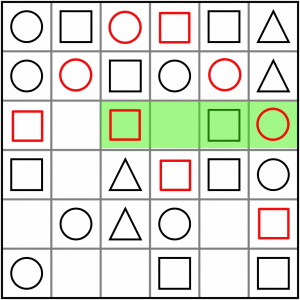
And here’s an ABxB. As we fill out the cells, the simple patterns keep popping up again, so it’s worth keeping an eye out for them. This must be a circle.
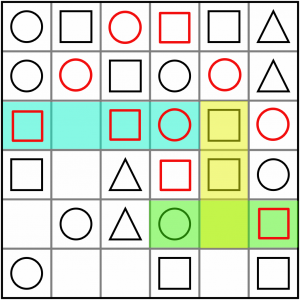
Let’s speed up a bit. We’ve got two patterns here. The blue is another ABxB, so that’s a circle. The yellow and green intersection is an AAx / AxC, so that has to solves to C - a circle in this case.
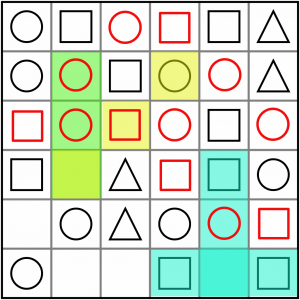
Nearly there. Here are two more. The yellow and green intersection is an AAx / ABx, so must solve to B. It’s a square.
The blue intersection is an AxA / ABx. It must solve to B, so it’s a circle.
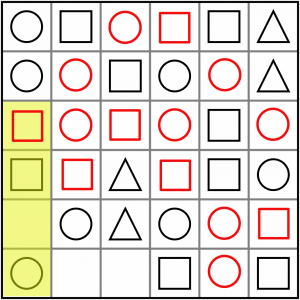
A quick AxCC here. It must be a circle.

Just two more to go. This is a new intersection: ABx / ACx. This must solve to A (square). If we put a B in here, we’d be creating an ACB run on the diagonal. if we a C in there, it would be an ABC on the vertical. So, it’s a square.
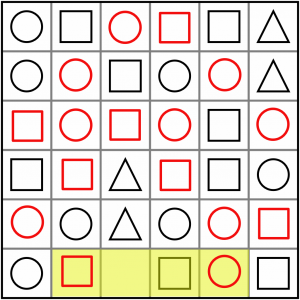
That just leaves us with a simple ABxB pattern along the bottom. It must be another circle.
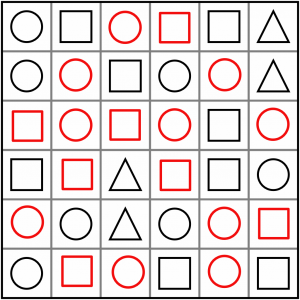
That’s it, we’ve completed the puzzle. And we didn’t even have to draw any triangles! I said it was an easy one. If you want to try some puzzles like this yourself, read on – there are some freebies to download below…
This was a very simple puzzle, but it shows the basic techniques used in solving Trilogy. Harder puzzles have far fewer starting symbols on the board, and fewer (if any) initial patterns. They require using more advanced logic, and notes in the cells as you eliminate possibilities.
Are you hooked? Ready to have a go at some Trilogy puzzles yourself? We’ve put together a sample pack of four grids for you – a couple of easy ones like the above example, and a couple that are more challenging. Download the PDF below. Solutions are included so you can check your results.
Click Here To Download The Taster PDF
Would you like even more Trilogy? We’ve got you covered!
Puzzle Weekly Presents: Trilogy is a collection of 120 puzzles set over seven levels of difficulty. Grab a copy here.
Got a Kobo? We’ve got Trilogy puzzles for that too! Trilogy for Stylus Devices presents 100 puzzles over five levels, and is designed especially for Kobos with stylus. Find out more here.
Brand new to Puzzle Weekly, Hitori makes its debut today. It's quick to learn and great fun to solve – we hope you like it. As if that wasn't enough, we've also got Star Battle and Yagit, plus all the usual features including:

On World Architecture Day we are celebrating the mathematical underpinnings of building. And doing lots of lovely new puzzles, of course! This week we've got Number Cross, Binairo, Sudoku, and all the usual features, including:

Arrows is a logic puzzle in which you must place arrows outside the grid according to certain rules.
In a hurry? Jump to: Rules / Tips / Worked Example / Download Puzzles / Books
Here’s what a small Arrows puzzle looks like:
Your task is to place arrows in the empty boxes around the grid in such a way that each box contains a single arrow that points to at least one number in the grid. The numbers tell you how many arrows point to that cell.
Here’s what the earlier example looks like once solved:
Solving these puzzles is all about elimination. They can seem daunting at first (especially larger ones), but by taking a methodical approach and applying logic, we can always reach the correct solution.
Before we get into specific tips, here are some useful things to bear in mind about Arrows puzzles.
We can attack a puzzle on two fronts: by looking at the numbers and trying to work out from which directions the arrows must point at them, or by looking at the arrow cells and working out where they must point. Considering that most numbers have eight potential arrows pointing at them, but any given arrow cell only has three possible directions in which it can point, we’re better off working from the arrows inwards. If we can eliminate two out of three directions that an arrow could point, we can complete the arrow cell.
Here are some tips to help you get started with solving Arrows puzzles. In a moment, we’ll work through a puzzle from start to finish and put these into practice.
Let’s put some of the tips above into practice and work through a puzzle from start to finish.
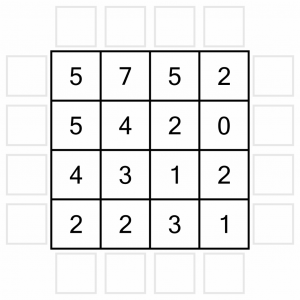
This is the puzzle we are going to work through. We’re using a very simple puzzle here as anything larger would make this walk-through way too long. Being an easy puzzle, we are provided with clue numbers in all the cells. Harder levels have fewer clues.
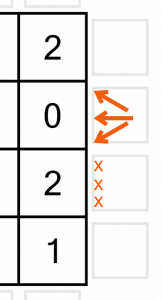
Before we get started, a quick word on notation. As we’ll be eliminating possible arrow directions, we need a consistent way of doing so. Any given cell has three possible arrow directions: up / down / horizontal for the sides, and left / right / vertical for the top and bottom. Here we have the three possible side arrows shown. As we eliminate possible placements, we can place an X in the relevant position to rule it out.
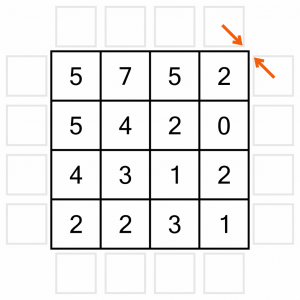
The very first thing we can do on any puzzle is eliminate ‘illegal’ arrow positions in the corners. The rules state that arrows must point towards at least one number. That means that the arrows shown here are not acceptable as they don’t point at any numbers….
…so we can put Xs in all those positions to rule them out. It’s not strictly necessary, but it makes things easier later on.
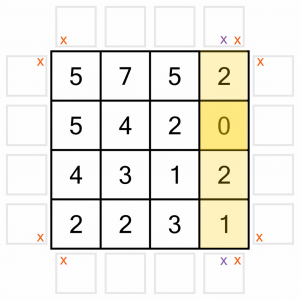
Now let’s look at the 0. No arrow can point to it, so we can add in more Xs as we eliminate all the positions that could point to it. That means no vertical arrows in the column the 0 is in. I’ve eliminated those with the purple Xs.
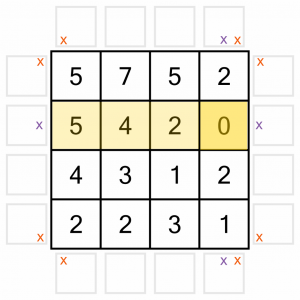
It also means no horizontal arrows for the row the 0 is in, so we eliminate those with Xs too.
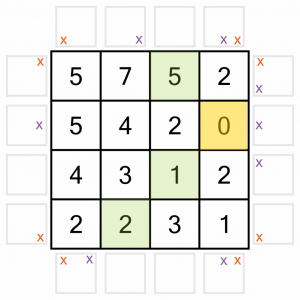
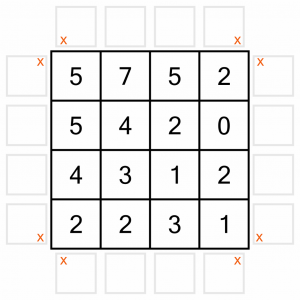
And of course we mustn’t forget the diagonals. That gives us four more arrow positions to eliminate.
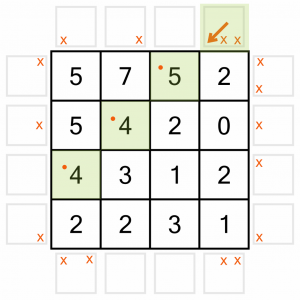
With all those eliminations, we’ve got some places to put some arrows on the board. We’ll start at the top. With the vertical arrow and diagonal right arrows not an option, we know we must place an arrow going diagonal left, pointing at the cells highlighted here.
At this point we are going to add another kind of note to the board. Each of the three highlighted cells now has an arrow pointing at them, so we’ll mark each one with a dot. This will help us keep track of which cells are ‘complete’, which will be essential later on.
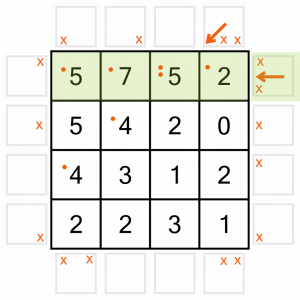
Working around clockwise, we can place a horizontal arrow pointing to this row. Again, we add a dot to each of the cells it’s pointing to.

Still going clockwise, we have another placement here. And again, we add dots to all the numbers the arrow is pointing to.
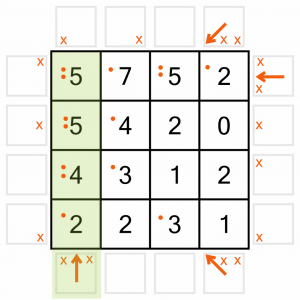
And lastly for now, we can put in this vertical arrow.
So where next? We’ve used up all of the known placements, so how can we eliminate any more? We’re going to have to find some constrained numbers – those with limited placement opportunities that can help us out.
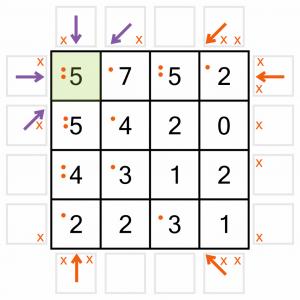
We know that numbers on the main diagonals are already more constrained than the other numbers on the board, as they can only have a maximum of six arrows pointing at them, not eight. The largest number on a main diagonal is this 5. It’s already got two arrows pointing to it, so it needs three more. There are four more potentials, but we don’t know which three are the ones we need to use.
Note: Although we don’t know which of those four arrows will be used, we do know that at least one of the ones on the diagonal will have to be used. Had this 5 been deeper in the board and not in a corner, that information could have allowed us to add a dot to all the other numbers on the same diagonal, which may have helped us move forward. In this puzzle, with the 5 being in the corner, it’s no help, but this is an essential technique when working on harder level puzzles.
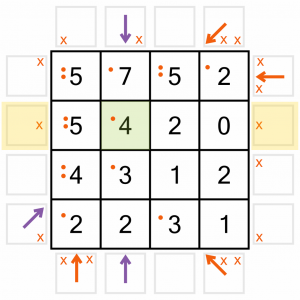
The next largest number on a main diagonal is this 4. It’s already got one arrow pointing to it. Out of the remaining five possibles, two have been eliminated (highlighted here in yellow). We need three arrows, and lo and behold there are only three places to put them!
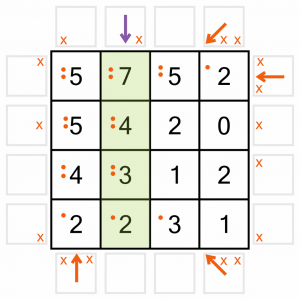
We’ll draw them in one at a time, taking care to add dots to our number tallies as we go. First the top one…
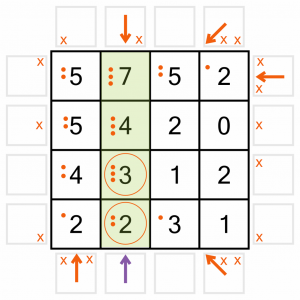
…then this bottom one. That’s ‘completed’ two of the numbers in this column. The dot tallies let us see at a glance that the 2 and the 3 both have the requisite number of arrows pointing at them. We’ll draw circles around those numbers to remind us we can’t point any more arrows at them. That will allow us to eliminate more potential arrow positions in a moment, but first…
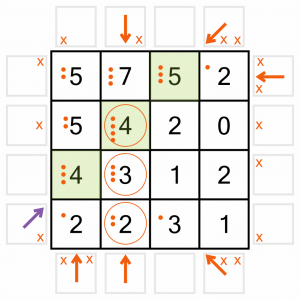
…we’ll just draw in that last arrow for the 4. Adding the dots confirms the 4 is now complete, so we can circle it.
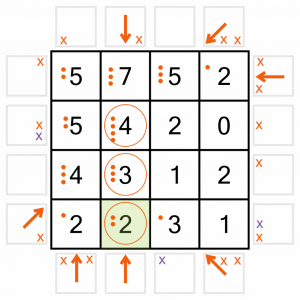
Now we can use these three newly circled cells to eliminate more arrow positions. The 2 gives us three new eliminations (purple Xs). I haven’t drawn in the one to the left of the row as that arrow is already placed, so we don’t need it.
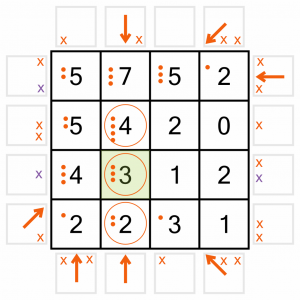
The completed 3 also eliminates three arrow positions (purple Xs).
We know the 4 doesn’t eliminate anything because we already used up all the remaining positions.
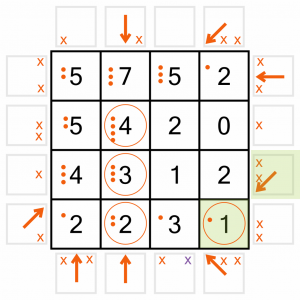
We’re really motoring now. We’ve got lots more arrows we can place. Going around the board clockwise again, we’ll start with this diagonal. That completes the 1 in the corner, which in turn allows us to eliminate an arrow position on the diagonal (purple X).
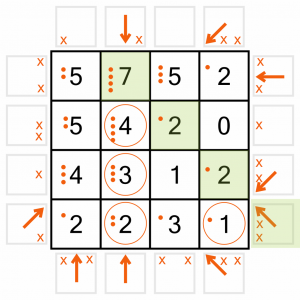
This next new arrow puts three more dots on the board…
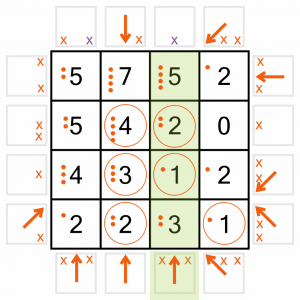
…and this one completes two numbers, which in turn give us two new eliminations.
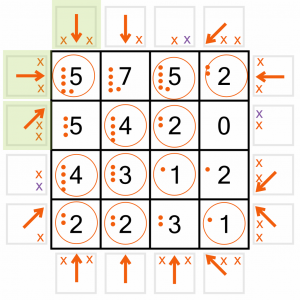
We can place all three arrows up in this corner. They complete several new numbers, which in turn eliminate further positions (again, the purple Xs).
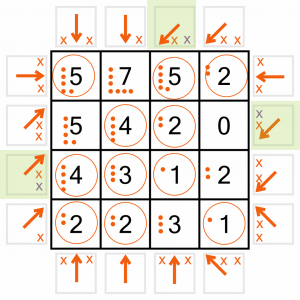
We know where to place the final three arrows. I’ve added the dot tallies to the numbers, but there’s no need to circle them, we’re all done!
Phew! How did you get on? Did you race ahead and finish before the end of the example? If you want to have a go and try some more, including some harder puzzles, there’s a taster pack to download below.
This was an easy puzzle, but it shows the basic techniques used in solving. Harder puzzles have fewer clue numbers, and require using more advanced logic like partial-elimination when you know the direction of an arrow (horizontal, vertical or diagonal), but not from which side it originates.
Ready to try some puzzles yourself? We’ve put together a sample pack of four grids for you – a couple of easy ones like this example, and a couple that are more challenging. Download the PDF below. Solutions are included so you can check your results.
Click Here To Download The Taster PDF
Ready for even more Arrows? We’ve got you covered!
Puzzle Weekly Presents: Arrows is a collection of 120 puzzles set over seven levels of difficulty. Grab a copy here.
Got a Kobo? We’ve got Arrows puzzles for that too! Arrows for Stylus Devices presents 100 puzzles over five levels, and is designed especially for Kobos with stylus. Find out more here.
Sort out your horizontals from your verticals and diagonals, with Line Segment, in this week's free Puzzle Weekly. We're also path-finding with Hidato. Plus: all the usual features, including:

Hitori is a logic puzzle that, like Sudoku, uses numbers as symbols but does not involve arithmetic.
In a hurry? Jump to: Rules / Tips / Example / Download Free Puzzles / Hitori Books
The objective is to cross out certain numbers such that each column and row contains no more than one of any given number.
Here’s what a small, simple Hitori puzzle looks like:
Your aim is to cross out, or shade, certain cells according to the following rules:
Here’s what our earlier small example puzzle looks like, once completed:
Hitori puzzles are solved with a combination of elimination and logical deduction. When solving, it helps if we remember we are not only looking for cells that we know must be shaded, but that we can also determine certain cells that must be left unshaded. These can be just as useful to discover as we work our way through the board.
Here are some tips for solving. Below, we’ll put them into practice and work through a complete puzzle from start to finish.
Interestingly, contrary to puzzles like Sudoku, you can’t tell you’ve finished a Hidato puzzle simply by filling in a final missing digit. You have to work out when you’re done by constantly checking if there are any remaining duplicate digits. So it’s always worth double-checking before you declare your puzzle complete and check your result!
Now that we know how to attack Hitori puzzles, let’s work through an example from start to finish.
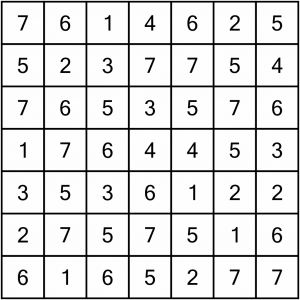
This is the puzzle we are going to solve. It’s a Level 1 puzzle; 7x7, easy to solve but sufficient to demonstrate the common techniques we use.
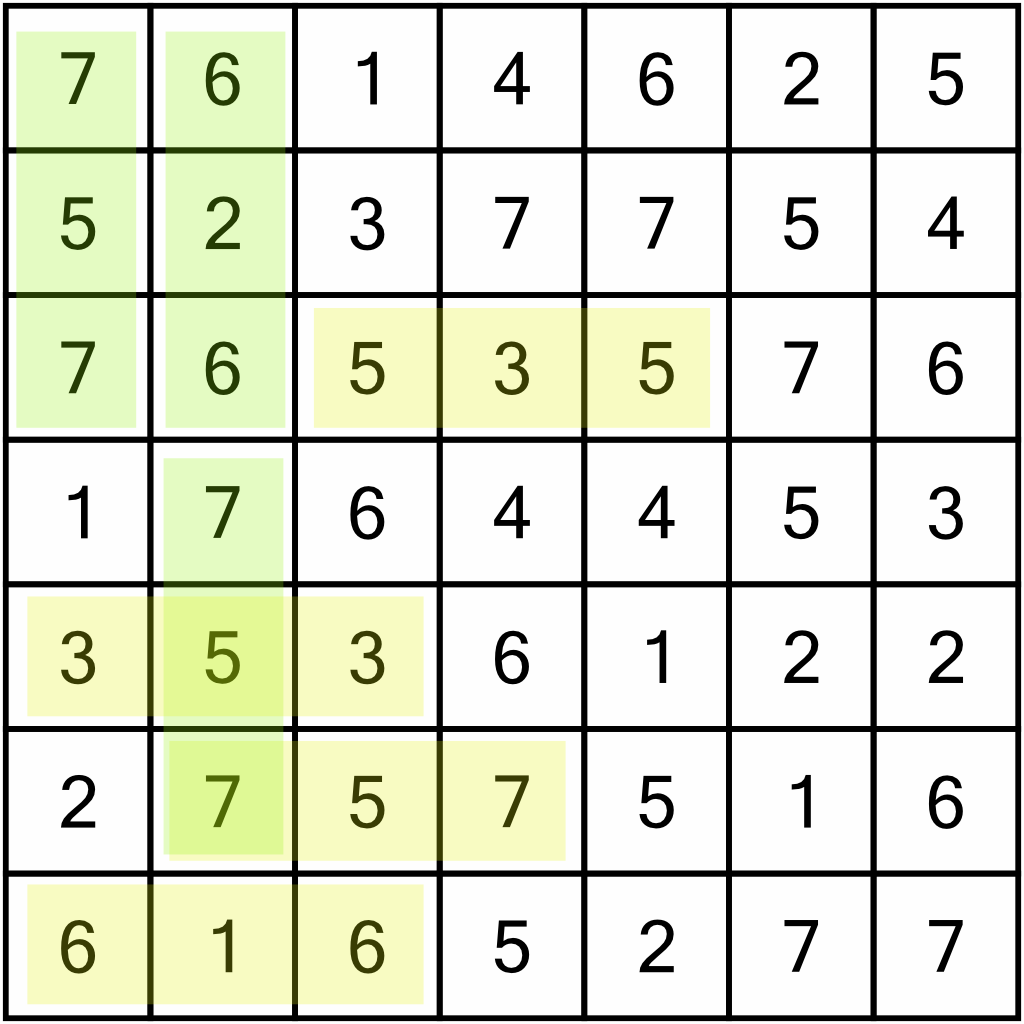
We’ll begin by looking for the easy patterns. There aren’t any ‘3 in a row’ patterns here, and neither are there any “2+1” patterns. There are quite a few “XYX”s though, which are shown here in yellow for rows and green for columns. We know that in each of these cases, we can circle the middle digit – it must be kept, because we know we’ll have to eliminate (shade) at least one digit immediately adjacent to it. We don’t know which will be shaded (and indeed it could be both), but it doesn’t matter, at least one will be and as we cannot have two shaded cells next to each other, the middle cell can’t be shaded so must be circled.
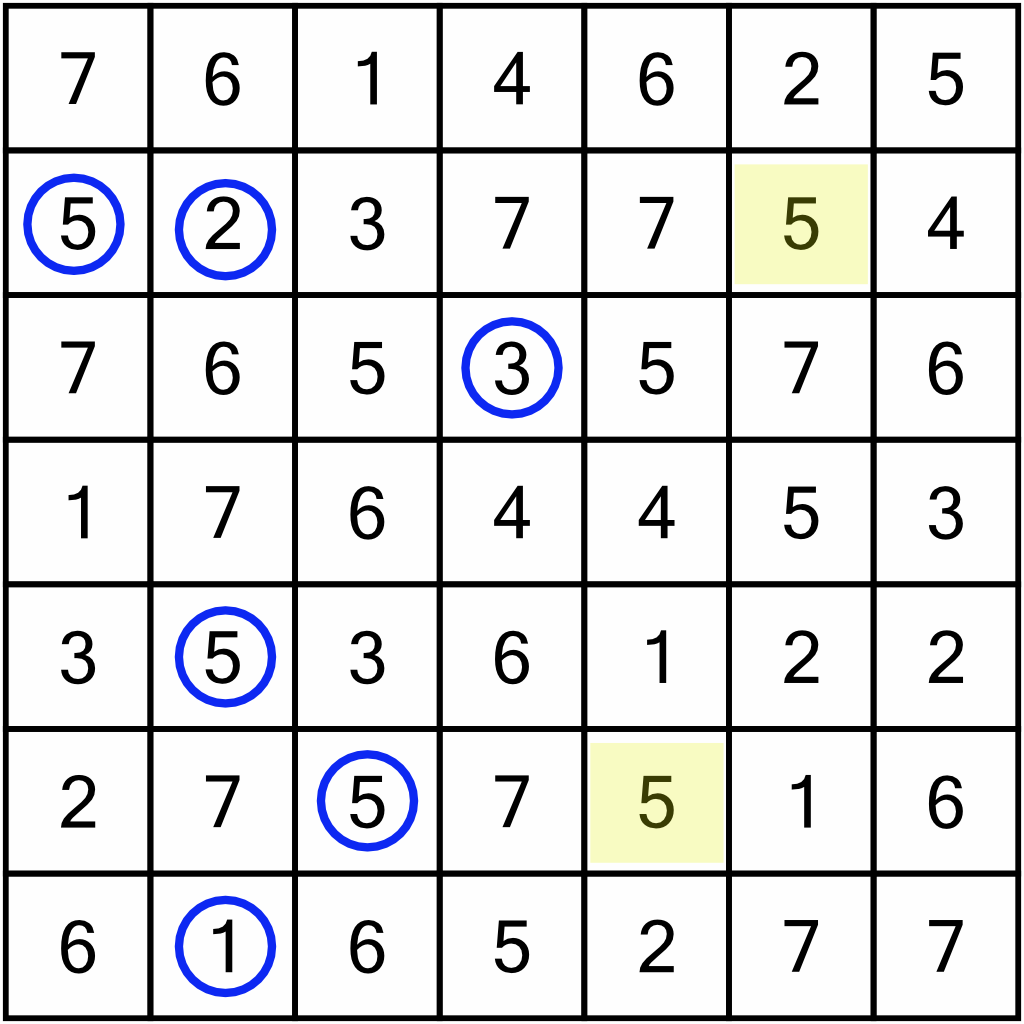
Now we can look along each row with circled digits, and see if there are duplicates of those digits. If there are, they can be eliminated. In row 2 we are looking for any other 5s and 2s. There is a 5, so we will be able to eliminate it.
Row 6 also has a duplicate 5 that we can take out.
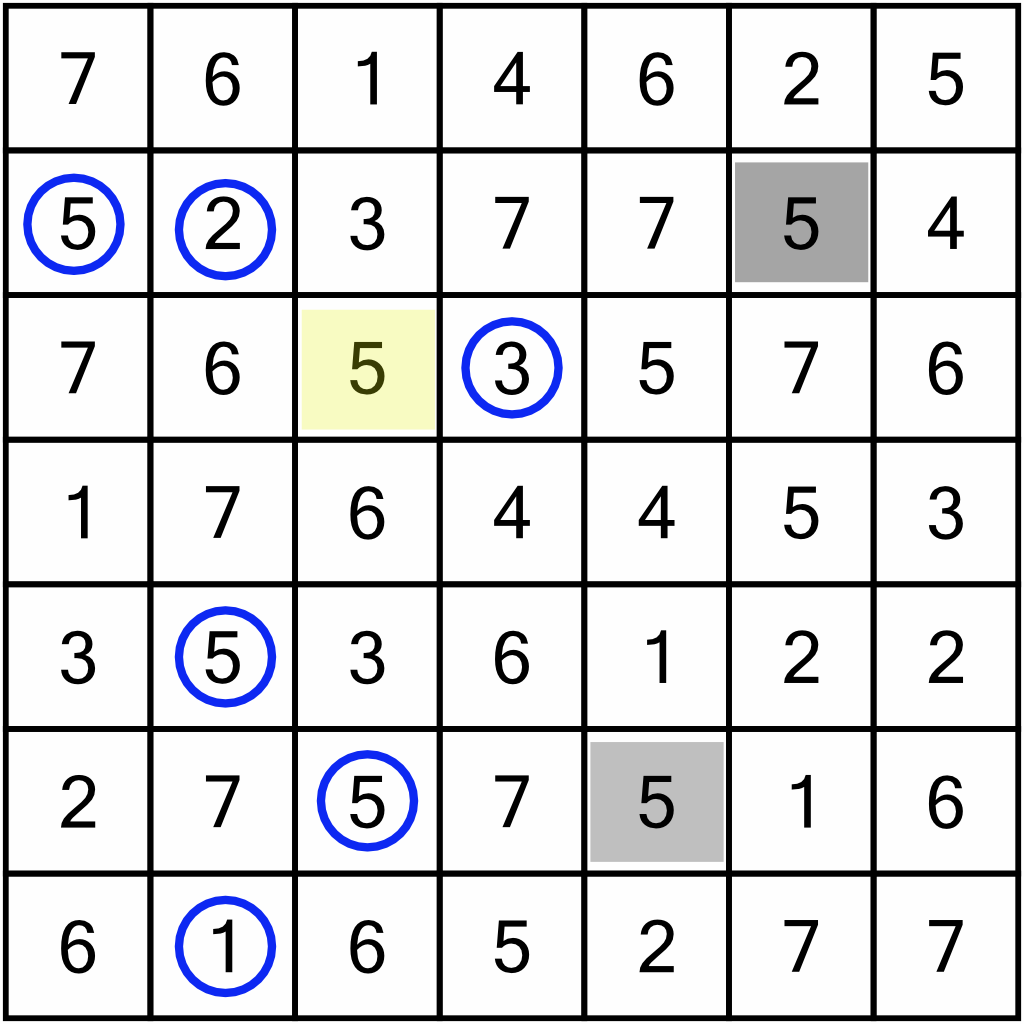
We’ll do the same for the columns. In column 3, there’s a duplicate 5, so that can be shaded in.
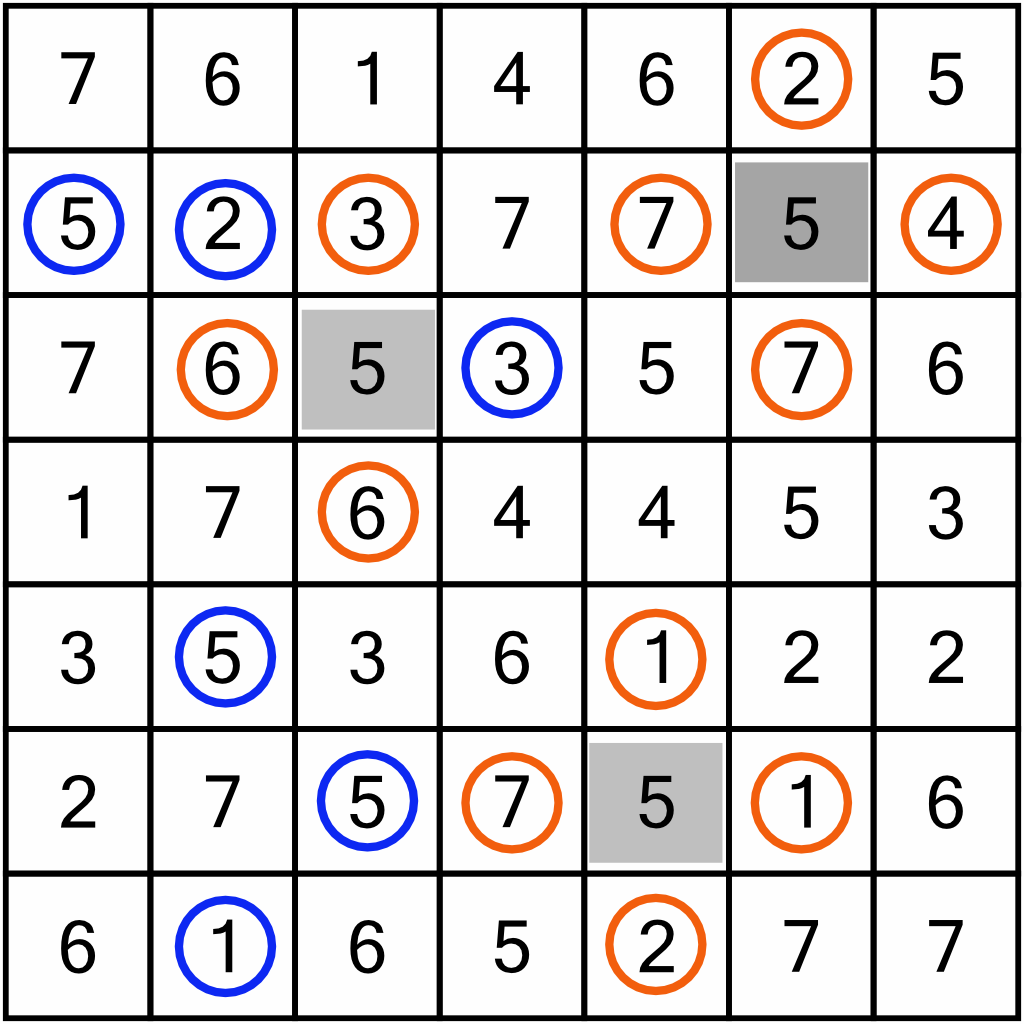
As we know that we can never have two shaded squares orthogonally adjacent, we can circle the digits immediately above, below, to the left, and to the right of those we have shaded in. They must be kept. We’ve circled these here in orange.
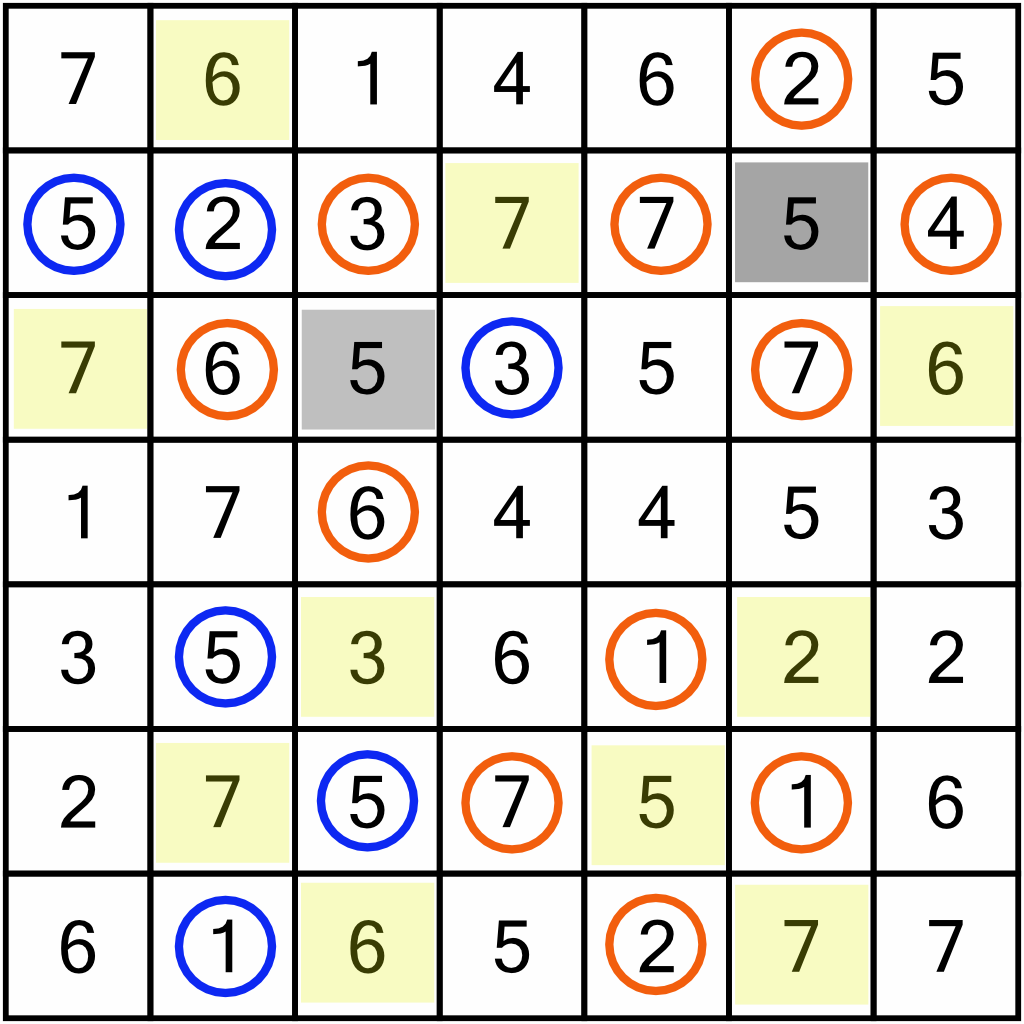
With eleven new circles on the board, we can go back through the rows and columns looking for duplicates we will be able to remove. For example, in column 2 we’ve a newly circled 6, so we can eliminate the duplicate 6 right at the top. Between all the rows and columns, that lets us eliminate ten new cells (shown here in yellow).
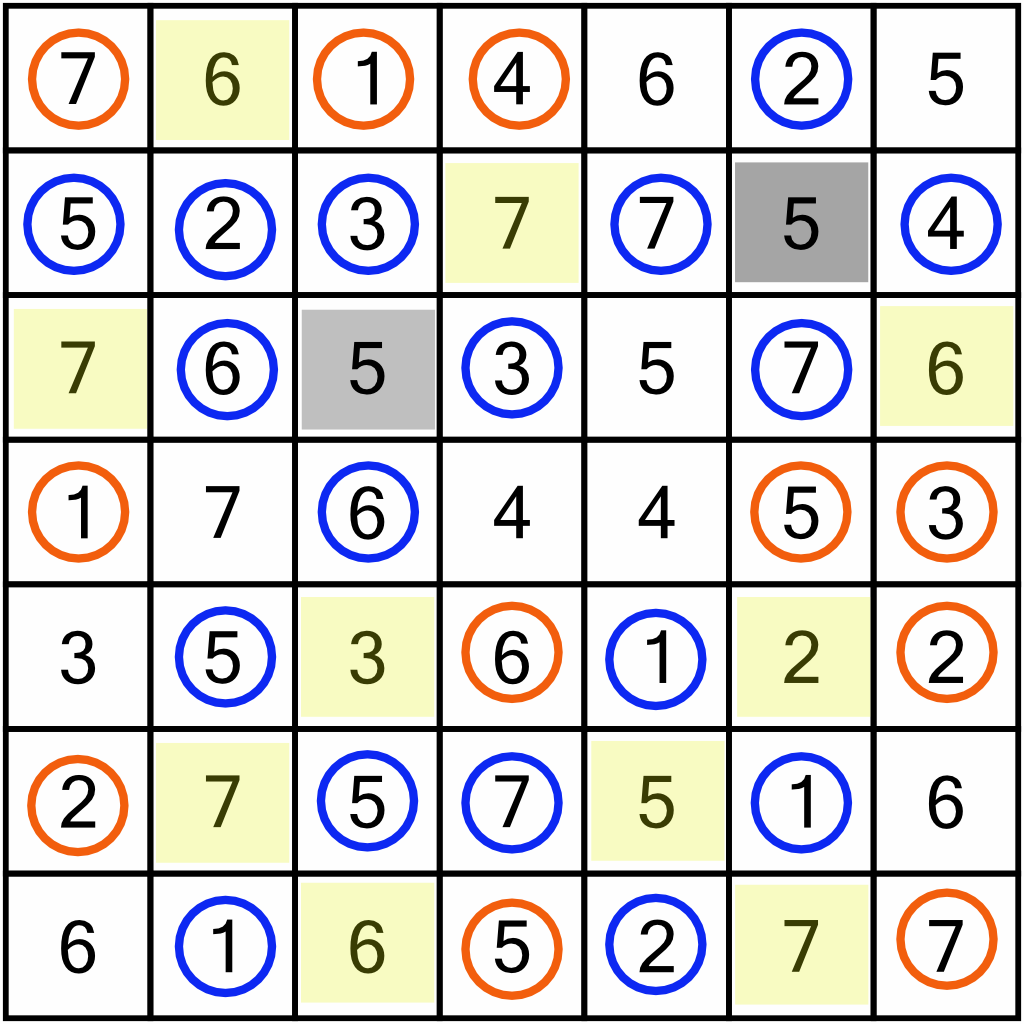
Now we repeat our earlier check, circling the cells above, below, and to the left and right of the newly eliminated ones. That gives us lots of new circles (shown here in orange)…
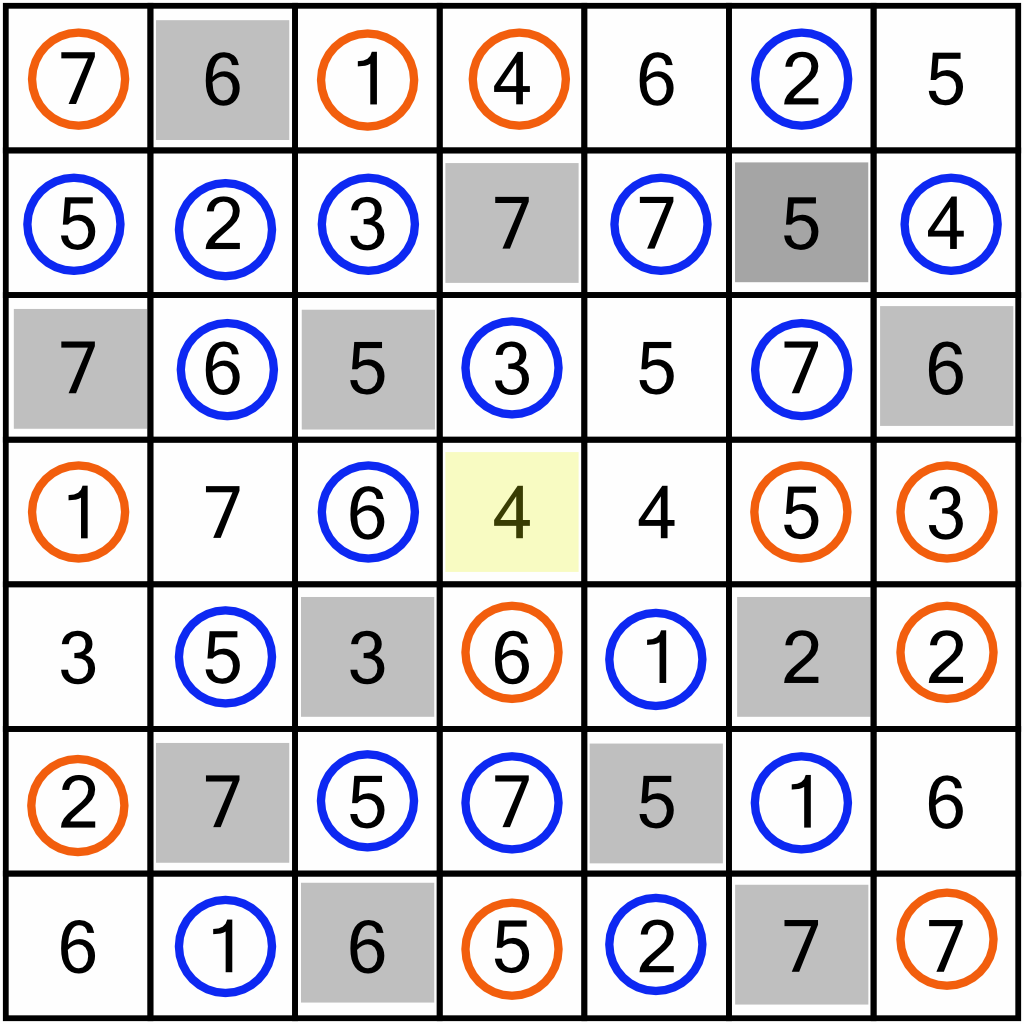
…and of course, we can use those to further eliminate doubles in the rows and columns. In fact, despite all those nice new orange circles, we can only eliminate one new cell – the 4 right in the middle.
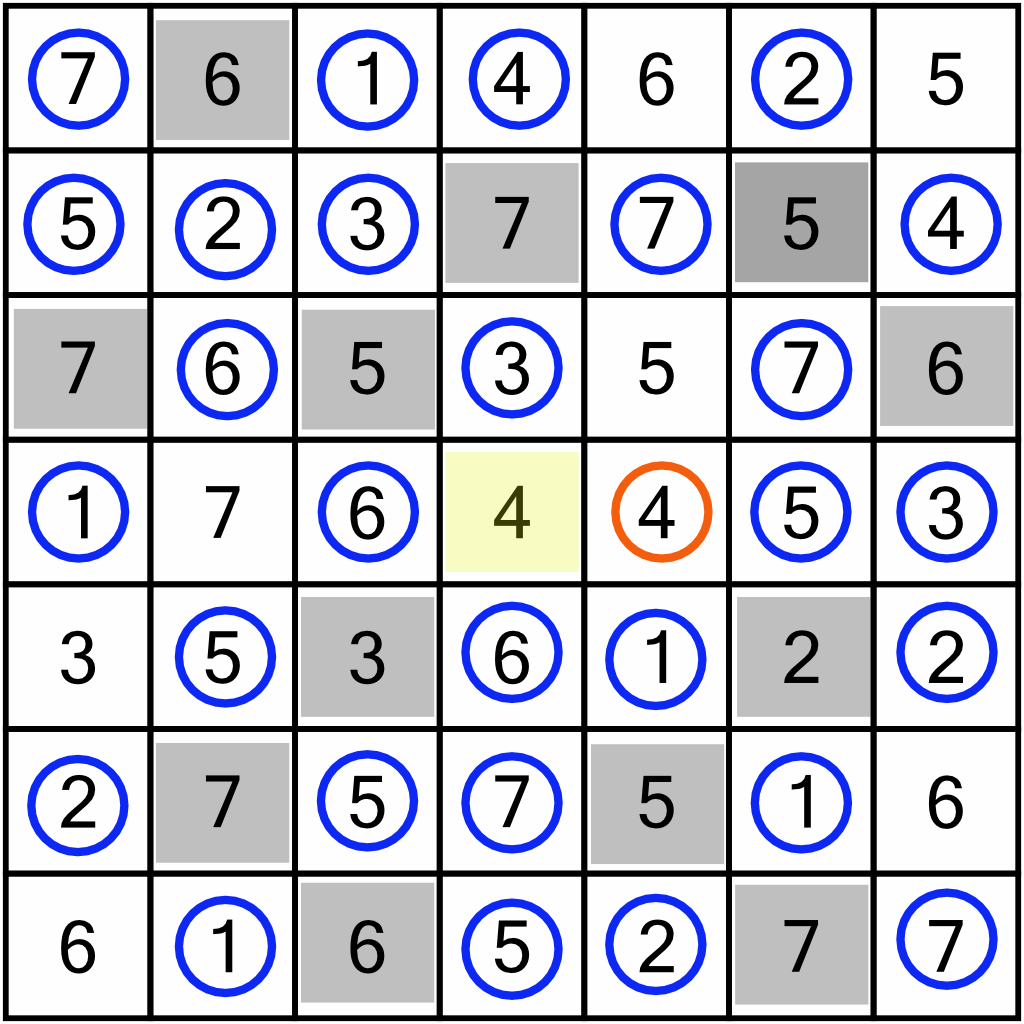
That 4 lets us circle the 4 immediately to its right.
We’ve reached a dead-end with the rippling of these eliminations; the four newly circled in orange don’t let us eliminate anything new, so where next? Let’s take stock of the board…remember, we only know that we’ve completed the puzzle once we are sure there are no duplicate numbers in any rows and columns.
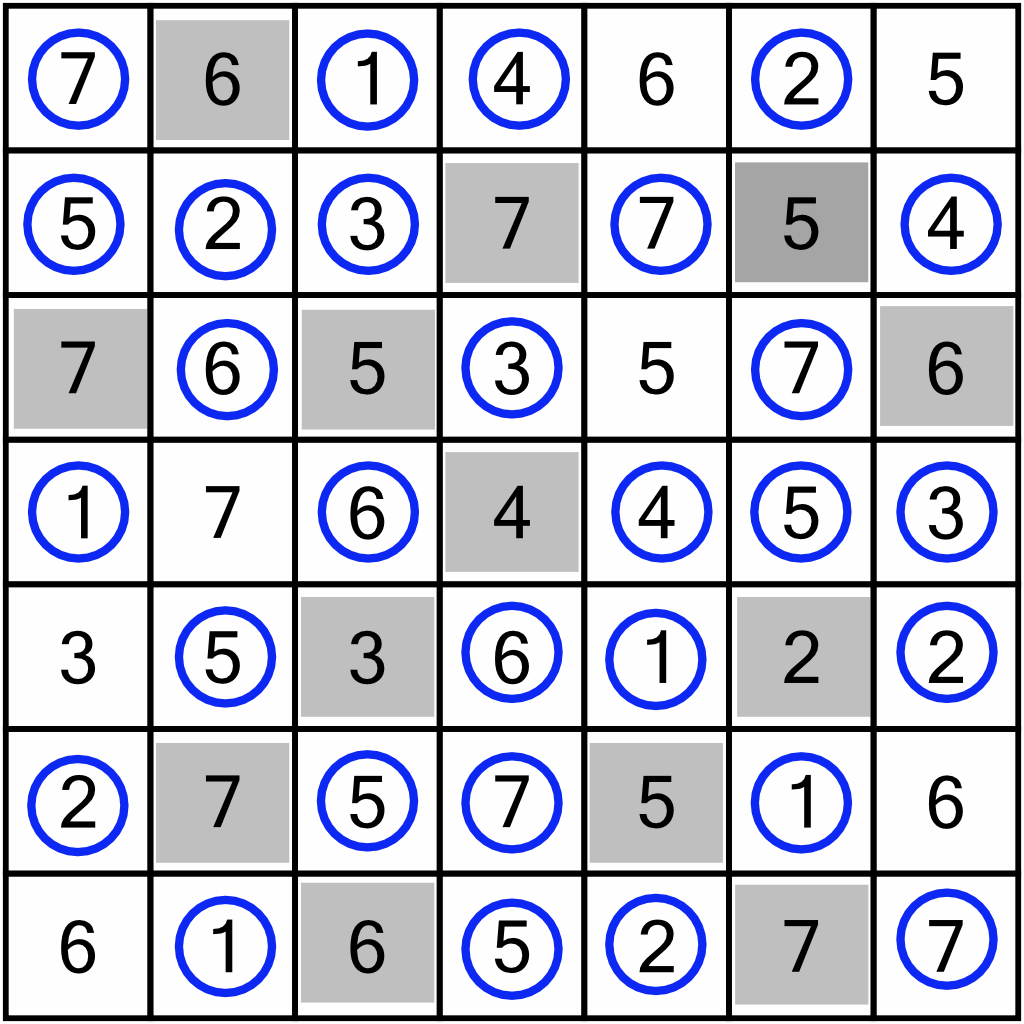
In fact, there are no more duplicates in any row or column, so we’ve completed the puzzle. I said it was an easy one!
Of course, being a Level 1 puzzle, that was pretty simple to solve. Everything rippled out and we could just use basic techniques. As puzzles get harder, you’ll need to take into account the rule about non-shaded cells making a single contiguous unit. Even harder puzzles will require chaining logic to determine which cells to keep and which to eliminate.
Ready to try some puzzles yourself? We’ve put together a sample pack of four grids for you – a couple of easy ones, and a couple that are more challenging. Download the PDF below. Solutions are included so you can check your results.
Click Here To Download The Taster PDF
Ready for even more Hitori? We’ve got you covered!
Puzzle Weekly Presents: Hitori is a collection of 120 puzzles set over seven levels of difficulty. Grab a copy here.
Got a Kobo? We’ve got Hitori puzzles for that too! Hitori for Stylus Devices presents 100 puzzles over five levels, and is designed especially for Kobos with stylus. Find out more here.
Happy equinox! It doesn't matter if you prefer solving your puzzles in the light or the dark, you have equal amounts of each today...or do you? This week, Tetromino is back. Also, Tents! Plus, other puzzles that don't begin with 'T', including:

Exercise those "little grey cells" with 28 brand-new puzzles as we celebrate the birthday of the greatest detective novelist who ever lived! This week's challenges include Meadows, No Four in a Row, and all the usual features, including:

Grab those old 3D glasses from the junk drawer, they just might help with this week's dimensionally-challenging teaser, Skyscrapers. As if building upwards wasn't enough, we're building horizontally too, in Hashiwokakero. Plus, all the usual features, including:
