If you want to try your hand at Suguru but don’t know where to start, you’ve come to the right place.
In a hurry? Jump to: The Grid / Rules / Solving Suguru / Video Tutorial / Download Free Puzzles

Love Suguru? Indulge your puzzle passion with book from our fab collection. From pocket size to jumbo yearly-collections, we've got Suguru for all situations! Click here to see the range...
Assuming no prior knowledge of this captivating puzzle, we’re going to look at how the grid works, cover the simple rules, then dive in to the techniques we can use to solve Suguru. Let’s get started, by looking at…
Suguru grids do not have to be square. It’s possible to make puzzles of various shapes and sizes. Here at Puzzle Genius we are purists at heart, and we prefer the perfection of the square form. But we do publish puzzles of differing sizes — and of course — different levels. To start us off, here is an easy 1-star grid, six cells across by six down. It’s taken from our popular Pocket Suguru series:
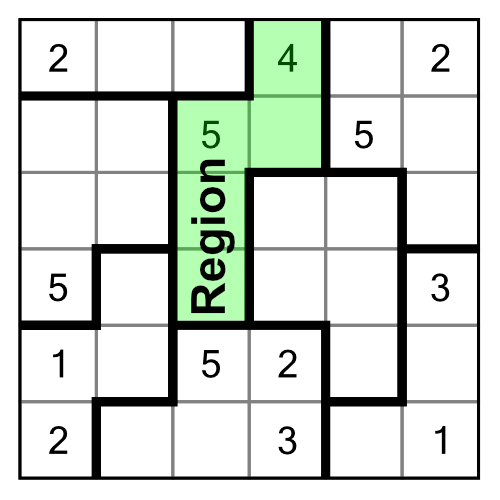
Like a Sudoku grid, we have some numbers filled in to start us off (clues). But that’s where the similarity ends. You’ll see there are no columns, rows, or blocks here. Instead we have regions.
There is no fixed number of regions in a Suguru grid, and the regions are not of fixed sizes. Indeed those two variables, along with the number of pre-filled clue cells, contribute to the difficulty of the puzzle.
Prefer to learn visually? Skip down to our video tutorial...
Suguru’s rules are very simple. In fact there are only two:
Looking back at our example grid, we note that the green shaded region has five cells, so it must contain all the digits from 1 to 5.
When we talk about neighbouring cells in suguru, we mean any cell that touches another horizontally, vertically, or diagonally. Here’s our example grid again:
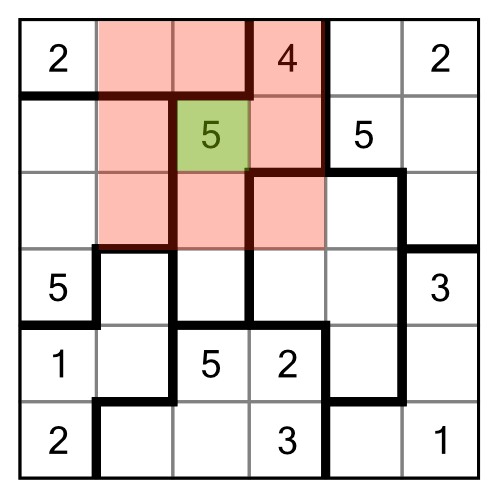
If we consider the green cell 5, all the cells shaded red are its neighbours, so none of them can contain the number 5.
Just as with sudoku, we can always solve a Suguru puzzle with logic. Guessing is not a strategy.
Just like Sudoku, some cells in a Suguru puzzle are very easy to solve right off the bat with nothing more than a simple application of the rules.
Our puzzle has a an obvious place to begin. The region in the bottom right has four cells and is missing a 2 and a 4:
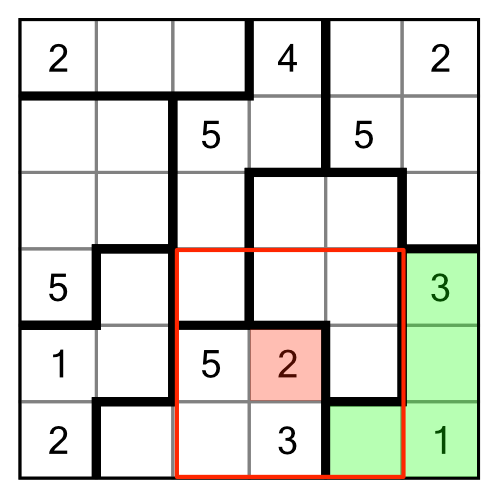
The presence of the 2 highlighted in red means we cannot put another 2 anywhere inside the red outline. That rules out one of our empty cells in the green region. Therefore the 2 has to go in the other one, in turn leaving only one place for the 4.
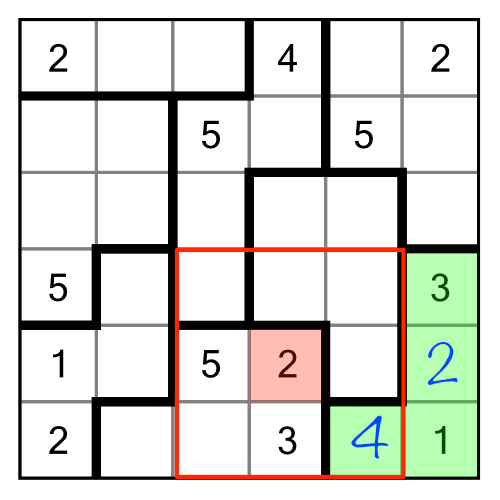
We can apply the same logic here:
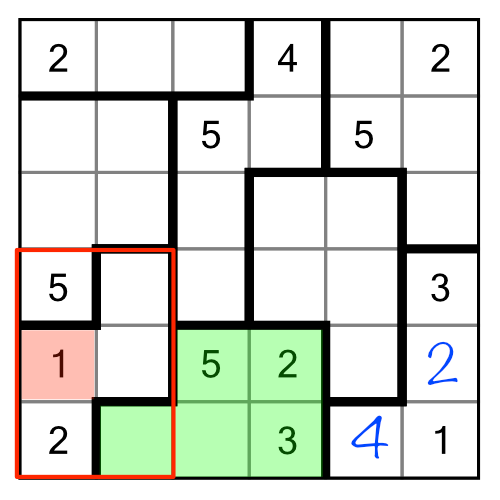
Our green region is missing a 1 and a 4. The red highlighted 1 rules out the left-most free cell in our region, leaving only one place it can go. The 4 must therefore go in the other one.
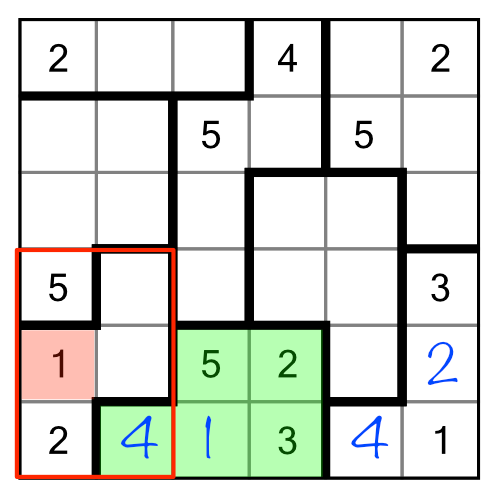
We can quickly fill in another couple of cells the same way:
The red highlighted 4 makes them easy to work out.
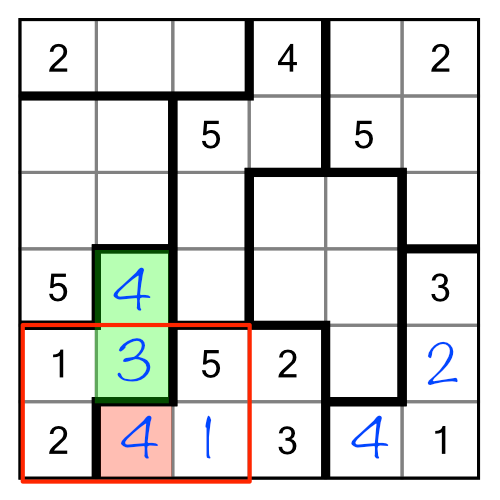
Once we’ve filled in the most obvious cells, we can move on to using an elimination strategy. Consider the region and cell highlighted:
At first glance this looks a little more complicated because now we have three cells within a region that we need to fill in. But actually we can apply the same logic to eliminate certain possibilities.
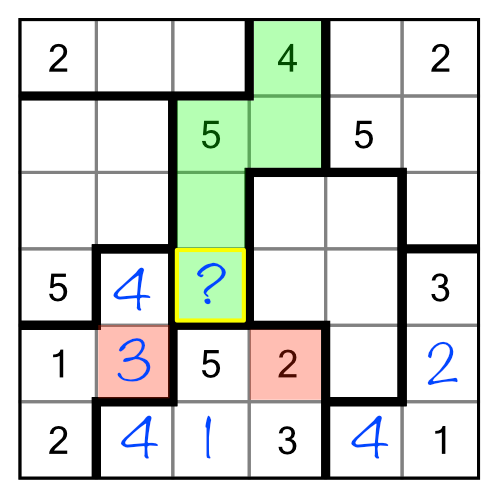
The red 3 and 2 prevent us from putting either of those numbers in our cell in question, which only leaves the 1.
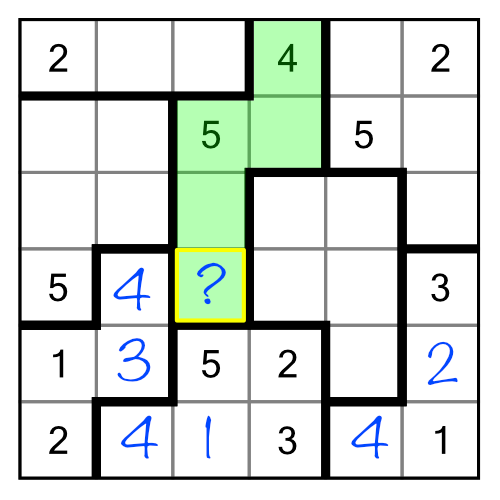
There’s another way we can use elimination, and it’s a bit more subtle. So far we’ve used pre-filled and solved cells to eliminate candidates. But in suguru we can also eliminate candidates based on implication. Consider the region with no numbers in it at all:
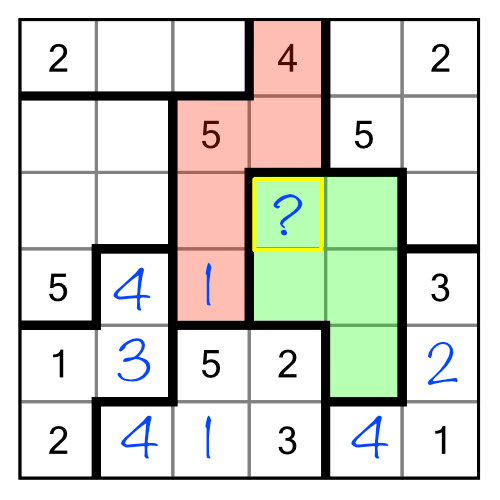
We know we need to find all the digits from 1 to 5 to fill this region. We can use the neighbouring (red) region to help us get started. The red region needs a 2 and a 3 in order to be completed. Given how our green region is partially surrounded by the red one, we can automatically eliminate 1, 2 and 3 as candidates for the cell with a “?” inside, and of course we can eliminate the 5 as well. Even if we didn’t already have the 1 and the 5 filled in inside the red region, we could still eliminate 1, 2, 3 and 5 based purely on implication. Putting any one of them into our “?” cell would make the red region impossible to solve! Therefore we can deduce that the only possible digit that can go there is 4.
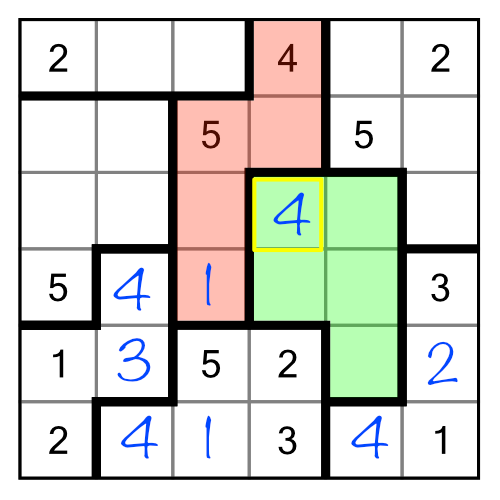
That’s a simple example of elimination by implication, but it’s a powerful technique that can be used to solve regions that at first glance appear to have no clues to go on. To see how powerful, here's an example from a different puzzle:

It looks like we have no clues at all to help us here, but of course that’s not true. There are certain things we do know. The green region has five cells, so requires the digits 1-5. The red region has four cells so needs 1-4. Elimination by implication tells us what the “?” cell contains. Given its position surrounded by the red region, it cannot contain a 1, 2, 3, or 4. If it did, it would be impossible to put any of those digits into the red region. Therefore it must contain a 5 - it’s the only option.
Looking back at our example puzzle, it can easily be solved now with simple elimination.
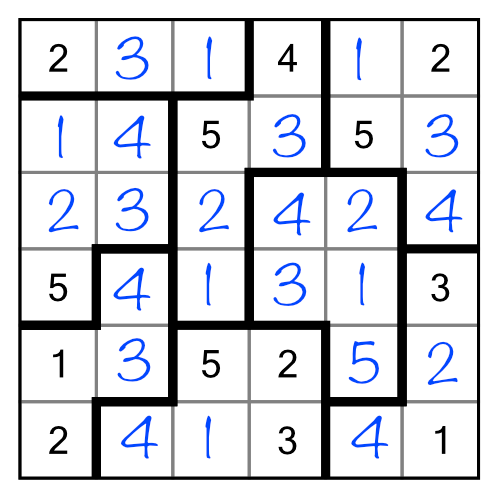
Just as with Sudoku, writing in small numbers to show candidates for a cell can be a useful thing to do — especially on harder grids. It can ease cognitive load and show you opportunities that might not be obvious otherwise. Consider this puzzle:

We’ve already solved the 5 in the green region based on elimination by implication. Where do we put the 1 and the 3 though? For now we don’t know, so we can write them in as little numbers:
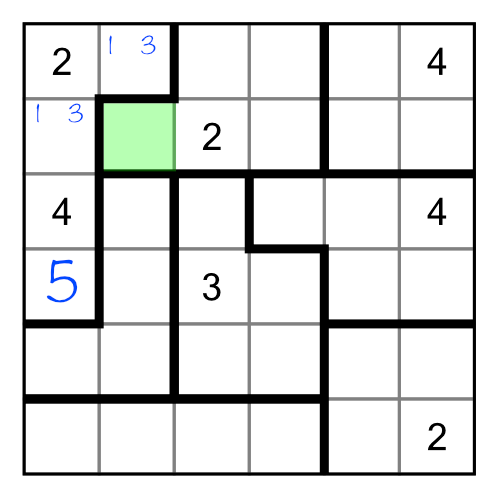
Now when we come to consider the green cell, we can immediately see that it cannot be a 1, 2, 3 or 4, so must be a 5. Yes, we could have worked this out without writing in the small numbers, but when working bigger puzzles with larger regions, taking notes like this can make it easier to spot answers as your notes will ripple out across the puzzle. When that happens, some combinations of notes will eliminate candidates in others, solving cells that then ripple back through your earlier notes.
Suguru is all about elimination. Elimination by implication can give you a quick ‘in’ to fill out some extra clue cells, helping you get started. Taking notes on harder puzzles will uncover hidden elimination opportunities.
If you find you get stuck in a puzzle, work through noting all possible candidates in the empty cells, and you will find pairs of candidates that, when combined, eliminate candidates from pairs of neighbours.
To help you practice, we’ve put together a set of easy level suguru puzzles you can print out, along with the solutions in a separate PDF. You can download both below.
If you enjoy Suguru and would like enough puzzles to keep you going for an entire year, then our A Year of Suguru books are for you. Large grids, and a daily rotation through five levels of difficulty mean you’ll have a fresh challenge every day of the year. Choose from Easy-Intermediate and Intermediate-Hard editions.
Right click or long-tap and Download Linked File or click or tap to open in a new window then choose Print from your browser.